|
Substituting numbers into formula, part 3 |
|
|
In this lesson we will learn how to substitute numbers into more complicated formulas. Rob uses real formulas and real examples from Science in this lesson. This lesson takes you to New Zealand where you will see Dolphins chasing boats and jumping out of the water, Mt Everest on a very cold day and to the Eiffel Tower in France.
The questions covered in this lesson are all real equations and real life examples: i) Using the formula $KE$ = $\frac{{mv}^2}{2}$, find the kinetic energy, $KE,\ $of a dolphin with a mass of 300kg and velocity of 15m/s. ii) Using the formula $F$ = $\frac{9}{5}^\circ{C}$ + 32, a warm day on Mt. Everest is -$10^\circ{C}$, convert this temperature into Fahrenheit. iii) Using the formula $t$ = $\sqrt{\frac{2h}{g}}$, calculate the time it takes a 2 pence to fall from the Eiffel Tower, $h$ = 325 and $g$ = 9.8 |
Straight Line Graphs |
Exam tip -- completing a table of values |
|
|
In this lesson we will learn a really quick and extremely easy way of completing a table of values for any straight line graph in the form of y = mx + c
The questions covered in this lesson are, make a table of values for the following straight line graphs i) $y$ = 2$x$ + 1 ii) $y$ = -3$x$ + 8 iii) $y$ = 3$x$ ${-}$ 2 iv) $y$ = -2$x$ + 7 |
Plotting straight line graphs from a table of values |
|
|
In this lesson we will learn how to plot any straight-line graph from a table of values. You will learn tips on accurately plotting your points and how to avoid common mistakes often made by students when drawing graphs.
The questions covered in this lesson are i) plot the graph of $y$ = 2$x$ +1 ii) plot the graph of y = -3$x$ + 5 |
Simplifying Expressions |
Simplifying Expressions Part 3 |
|
|
In this lesson we will learn how to simplify more complicated expressions containing 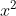 terms. terms.
The questions that are covered in this lesson are, i) simplify the expression  ii) simplify the expression ii) simplify the expression  . . |
|
Calculating average speed from a D/t graph part 1 |
|
|
There are 2 methods for calculating the average speed from a distance/time graph. In this lesson you will learn the first of these methods. Make sure that you also watch part 2 of this lesson to learn the other method. |
Calculating average speed from a D/t graph part 2 |
|
|
In this lesson we will learn the second method for calculating the average speed from a d/t graph. You must be able to calculate the gradient of any straight line graph before trying this lesson. If not then watch the following lesson in Algebra: Finding the gradient of a line.
|
Speed/distance/time questions - Common mistakes |
|
|
Thousands of students every year make this common mistake involving time when answering speed/distance/time questions. Rob will show you how to get your questions correct everytime. |
Calculating acceleration from a V/t graph part 1 |
|
|
There are 2 methods for calculating acceleration from a distance/time graph. In this lesson you will learn the first of these methods. You will also learn the units of acceleration. Make sure that you also watch part 2 of this lesson to learn the other method. |
Calculating acceleration from a V/t graph part 2 |
|
|
In this lesson we will learn the second method for calculating the acceleration from a v/t graph. You must be able to calculate the gradient of any straight line graph before trying this lesson. If not then watch the following lesson in Algebra: Finding the gradient of a line. |
Calculating the deceleration from a V/t graph |
|
|
In this lesson we will learn how to calculate the deceleration from a v/t graph. You must be able to calculate the acceleration from a v/t graph and find the gradient of any straight line graph before trying this lesson. If not then watch the following lessons in Algebra: Calculating acceleration from a v/t graph part 1/part 2 and/or Finding the gradient of a line. |
Calculating total distance travelled from a V/t graph |
|
|
In this lesson we will learn how to calculate the total distance travelled from a velocity/time graph. You must be able to calculate the area of a reactangle, triangle and trapezium before trying this lesson. If not then watch the following lessons in Geoemtry and measures: Calculating the area of a rectangle and/or calculating the area of a triangle and/or calculating the area of a trapezium. |
Estimating distance travelled under a curved V/t graph |
|
|
In this lesson we will learn how to estimate the total distance travelled from a curved velocity/time graph. You must be able to calculate the distance from a v/t graph before trying this lesson. You also need to be able to calculate the area of a reactangle, triangle and trapezium before trying this lesson. If not then watch the following lessons in Geometry and measures: Calculating the total distance travelled from a v/t graph and/or calculating the area of a rectangle and/or calculating the area of a triangle and/or calculating the area of a trapezium. |








